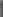

Spreadsheet Models for Managers
Getting Access to Spreadsheet Models for Managers
If  you use Excel to model businesses, business processes, or
business transactions, this course will change your life. You’ll learn how to create tools for yourself that will amaze
even you. Unrestricted use of this material is available in two ways.
you use Excel to model businesses, business processes, or
business transactions, this course will change your life. You’ll learn how to create tools for yourself that will amaze
even you. Unrestricted use of this material is available in two ways.
- As a stand-alone Web site
- It resides on your computer, and you can use it anywhere. No need for Internet access.
- At this Web site
- If you have access to the Internet whenever you want to view this material, you can purchase on-line access. Unlimited usage. I’m constantly making improvements and you’ll get them as soon as they’re available.
To Order On Line
| Order "Spreadsheet Models for Managers, on-line edition, one month" by credit card, for USD 69.95 each, using our secure server, and receive download instructions by return email. |
| Order "Spreadsheet Models for Managers, on-line edition, three months" by credit card, for USD 199.00 each, using our secure server, and receive download instructions by return email. |
| Order "Spreadsheet Models for Managers, downloadable hyperbook edition" by credit card, for USD 199.00 each, using our secure server, and receive download instructions by return email. |
To Order by Mail
Make your check payable to Chaco Canyon Consulting, for the amount indicated:
|
And send it to: Chaco Canyon Consulting 700 Huron Avenue, Suite 19C Cambridge, MA 02138 |
To use the course software you’ll need some other applications, which you very probably already have. By placing your order, you’re confirming that you have the software you need, as described on this site.
Spreadsheet Models for Managers
| Matrix multiplication examples | 2/8 Session Links |
Here are four examples of matrix products. In each case, the left side of the equality contains the two matrices that are being multiplied, and the right side shows the result. The Excel worksheet function that produces the matrix product of two matrices is mmult.
Of the four examples shown here, only one is a legal product if the order of the two matrix factors on the left side of the equality is reversed. The one that’s legal in reverse order is the lower left product. Try reversing the order, and notice that the result of the reversed-order product isn’t equal to the result shown. This is an illustration of the fact that matrix multiplication isn’t commutative.
Sometimes we want to form the product of three or more matrices. Using mmult, we must do this by first forming the product of two of them, then multiplying the result of that by the third, and so on, maintaining the correct order, of course. It doesn’t matter how you pair them, as long as you preserve the order, because matrix multiplication is associative.
In your add-in for this course, you’ll find a function MMMult, which can handle up to ten matrix factors.
Last Modified: Sunday, 03-Aug-2025 03:54:37 EDT
For many of you, matrix multiplication and array arithmetic are new ideas. It’s easy to get lost in the details of how they work and then forget about why we use them.
To keep a clear view of the forest and avoid focusing only on the trees, remember why we use matrix multiplication and array arithmetic. Briefly, we use them because we find that it’s very often helpful to decompose a problem into parts (analysis), then do calculations on the parts, and finally reassemble the final solution from the results of those partial calculations (synthesis).
Matrix multiplication and array arithmetic provide us with very convenient methods for performing those intermediate calculations on the parts. They’re the tools that make analysis and synthesis so powerful.